Content Domain III: Measurement and Geometry

The shape of the letter B is designed as shown below, consisting of rectangles and semicircles.

The overall height is dimensioned as h. The height of each outer semicircle is dimensioned as h over 2. The height of each semicircular void (one in the upper half of the letter and one in the lower half of the letter) is dimensioned as h over 6. The thickness of the spine of the letter is dimensioned as h over 6.

Which of the following formulas gives the area A of the shaded region as a function of its height h?

1. A = h²A equals h squared times the quantity 1 over 6 plus pi over 18
2. A = h²A equals h squared times the quantity 1 over 6 plus pi over 9." />
3. A = h²A equals h squared times the quantity 1 over 6 plus 2pi over 9." />
4. A = h²A equals h squared times the quantity 1 over 6 plus 5pi over 18." />

Correct Response and Explanation (Show Correct ResponseHide Correct Response)

A. This question requires the examinee to apply the concepts of perimeter, circumference, area, surface area, and volume to solve real-world problems. The total area of the letter B can be viewed as the area of an h × h times h over 6 rectangle plus the area of a circle with radius h over 4 minus the area of a circle with radius , or + π − π.h over 12, or h squared over 6 plus pi times the square of h over 4 minus pi times the square of h over 12. This simplifies to + − h squared over 6 plus pi h squared over 16 minus pi h squared over 144 and further to h²h squared times the quantity 1 over 6 plus pi over 16 minus pi over 144 and h² and h²h squared times the quantity 1 over 6 plus 9pi over 144 minus pi over 144 and h squared times the quantity 1 over 6 plus pi over 18.

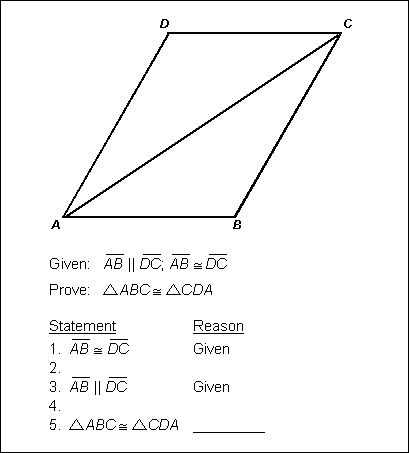
The figure is a parallelogram, ABCD. The angles at A and C are acute, and a diagonal is drawn from A to C. The proof reads as follows.
Given: Line segment AB is parallel to line segment DC, line segment A B is congruent to line segment DC.
Prove: Triangle ABC is congruent to triangle CDA.
Statement 1. Line segment AB is congruent to line segment DC. Reason, Given.
Statement 2. Blank. Reason, blank.
Statement 3. Line segment AB is parallel to line segment DC. Reason, Given.
Statement 4. Blank. Reason, blank.
Statement 5. Triangle ABC is congruent to triangle CDA. Reason, blank.

In the proof above, steps 2 and 4 are missing. Which of the following reasons justifies step 5?

1. AAS
2. ASA
3. SAS
4. SSS

Correct Response and Explanation (Show Correct ResponseHide Correct Response)

C. This question requires the examinee to analyze formal and informal geometric proofs, including the use of similarity and congruence. The side-angle-side (SAS) theorem can be used to show that ΔABCtriangle ABC and ΔCDAtriangle CDA are congruent if each has two sides and an included angle that are congruent with two sides and an included angle of the other. In the diagram AB and DCline segment AB and line segment DC are given as congruent, and the missing statement 2 is that AC is congruent to itself by the reflexive property of equality. The included angles ∠BAC and ∠DCAangle BAC and angle DCA are congruent because they are alternate interior angles constructed by the transversal ACline segment AC that crosses the parallel line segments AB and DCline segment AB and line segment DC. Thus ΔABC and ΔCDAtriangle ABC and triangle CDA meet the requirements for using SAS to prove congruence.

The vertices of triangle ABC are A(–5, 3), B(2, 2), and C(–1, –5)A left parenthesis negative 5, 3 right parenthesis, B left parenthesis 2, 2 right parenthesis, and C left parenthesis negative 1, negative 5 right parenthesis. Which of the following is the length of the median from vertex B to side AC?

1. 4
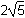2. 2 times the square root of 5
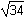3. the square root of 34
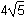4. 4 times the square root of 5

Correct Response and Explanation (Show Correct ResponseHide Correct Response)

C. This question requires the examinee to apply concepts of distance, midpoint, and slope to classify figures and solve problems in the coordinate plane. The midpoint of side AC where its median intersects is computed as 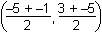= (–3, –1)left parenthesis negative the quantity 5 plus negative 1 over 2, the quantity 3 plus negative 5 over 2 right parenthesis equals left parenthesis negative 3, negative 1 right parenthesis. The distance from B(2, 2) to (–3, –1)B left parenthesis 2, 2 right parenthesis to left parenthesis negative 3, negative 1 right parenthesis is computed as d = 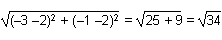.d equals the square root of the quantity open paren negative 3 negative 2 close paren the quantity squared plus open paren negative 1 negative 2 close paren the quantity squared equals the square root of the quantity 25 plus 9 equals the square root of 34.